All You Need To Know About Ratio And Proportion

Introduction To Ratio And Proportion

I assume the basic concepts of ratio and proportion have been studied by you in earlier classes, but in this post, we shall study ratio and proportion in more detail.

We shall also learn about continued proportion, mean proportion, componendo,  dividendo, alternendo, invertendo and their combinations, some applications on proportion.

Ratio

A ratio is a comparison of two or more quantities of the same kind by division.

If a and b are two quantities of the same kind (in the same units), then the fraction a/b is called the ratio of a to b. And it is written as a : b (read ‘a is to b’). Thus, the ratio of a to b = a : b.

For example, the ratio of 5 kg potato to 13 kg potato is 5 : 13.

Remember

1. Here a and b are called terms of the ratio; a is called the first term or antecedent and b is called the second term or consequent.
2. The consequent of a ratio cannot be zero.
3. If we multiply or divide both the terms of a ratio by the same non-zero number, the ratio remains unchanged.
4. If we multiply or divide both the terms of a ratio by the same non-zero number, the ratio remains unchanged.
5. A ratio must always be expressed in its lowest terms i.e. both the terms of the ratio must be co-prime.

Composition of Ratios

Compound Ratio

For two or more ratios, the ratio between the products of their antecedents to the product of their consequents is called compound ratio.

Example: For ratios a : b and c : d; the compound ratio is (a × c) : (b × d). For ratios a : b, c : d and e : f; the compound ratio is (a × c × e) : (b × d × f) and so on.

Duplicate Ratio

It is the compound ratio of two equal ratios.

Example: Duplicate ratio of a : b = Compound ratio of a : b and a : b = (a × a) : (b × b) = a² : b²

Triplicate Ratio

It is the compound ratio of three equal ratios.

Triplicate ratio of a : b = Compound ratio of a : b, a : b and a : b = (a × a × a) : (b × b × b) = a³ : b³

Sub-duplicate Ratio

For any ratio a : b, its sub-duplicate ratio is √a : √b

Sub-triplicate Ratio

For any ratio a : b, its sub-triplicate ratio is ∛a : ∛b

Reciprocal Ratio

For any ratio a : b; where a, b ≠ 0, its reciprocal ratio

\[\frac{1}{a}:\frac{1}{b}=b:a\]

Proportion

When two ratios are equal, they are said to be in ‘proportion’ and the four quantities composing them are said to be ‘proportionals’.

If a, b, c, d are in proportion we write it as a : b :: c : d.

Remember

Thus if, a : b = c : d, then a, b, c, d are proportional. The term a and d are called the extremes and b and c are called the means. d is called the fourth proportional, c is called the third proportional.

Product of extremes = Product of means

\[a:b=c:d\Rightarrow \frac{a}{b}=\frac{c}{d}\Rightarrow a\times d=b\times c\]

Continued Proportion

The non-zero quantities of same kind a, b, c, d, e, f, g, ……, are said to be in continued proportion if

\[\frac{a}{b}=\frac{b}{c}=\frac{c}{d}=\frac{d}{e}=\frac{e}{f}=\frac{f}{g}=……\]

If a, b, c are continued proportion, then a is called first proportional, b is called mean proportional of a and b, and c is called third proportional.

\[Here,\frac{a}{b}=\frac{b}{c}\Rightarrow {{b}^{2}}=ac\Rightarrow b=\sqrt{ac}\]

The mean proportion between two numbers is the positive square root of their product.

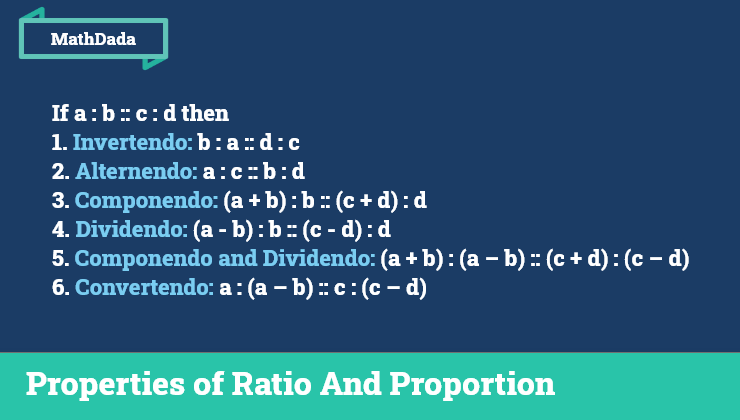
Properties of Ratio and Proportion

Quiz

[WpProQuiz 1]

Example 01

The following numbers k + 3, k + 2, 3k – 7 and 2k – 3 are in proportion. Find k.

Answer:

\[Here,~~\frac{k+3}{k+2}=\frac{3k-7}{2k-3}\]

\[\Rightarrow \left( k+3 \right)\left( 2k-3 \right)=\left( k+2 \right)\left( 3k-7 \right)\]

\[\Rightarrow 2{{k}^{2}}-3k+6k-9=3{{k}^{2}}-7k+6k-14\]

\[\Rightarrow {{k}^{2}}-4k-5=0\]

\[\Rightarrow \left( k-5 \right)\left( k+1 \right)=0\]

\[\Rightarrow k=5~~or~~k=-1\]

Example 02

What number must be added to each of the number 6, 15, 20 and 43 to make them proportional?

Answer:

Let the number must be added be x, then the new numbers are 6 + x, 15 + x, 20 + x and 43 + x.

Since they are proportional,

\[\left( 6+x \right):\left( 15+x \right)::\left( 20+x \right):\left( 43+x \right)\]

\[\Rightarrow \left( 6+x \right)\left( 43+x \right)=\left( 15+x \right)\left( 20+x \right)\]

\[\Rightarrow 258+6x+43x+{{x}^{2}}=300+15x+20x+{{x}^{2}}\]

\[\Rightarrow 49x-35x=300-258\]

\[\Rightarrow 14x=42\]

\[\therefore x=3\]

Example 03

The monthly pocket money of Ravi and Sanjeev are in the ratio 5:7. Their expenditure are in the ratio 3:5. If each saves Rs. 80 every month, find their monthly pocket money.

Answer:

Let monthly pocket money of Ravi is 5x and Sanjeev is 7x.

Then according to question

\[\Rightarrow \frac{5x-80}{7x-80}=\frac{3}{5}\]

\[\Rightarrow 25x-400=21x-240\]

\[\Rightarrow 25x-21x=400-240\]

\[\Rightarrow 4x=160\Rightarrow x=40\]

Therefore, Ravi’s pocket money = 5 × 40 = Rs. 200 and Sanjeev’s pocket money = 7 × 40 = Rs. 280.

Example 04

If b is the mean proportion between a and c, show that:

\[\frac{{{a}^{4}}+{{a}^{2}}{{b}^{2}}+{{b}^{4}}}{{{b}^{4}}+{{b}^{2}}{{c}^{2}}+{{c}^{4}}}=\frac{{{a}^{2}}}{{{c}^{2}}}\]

Answer:

Here, b is the mean proportion between a and c.

\[\therefore {{b}^{2}}=ac\]

\[L.H.S=\frac{{{a}^{4}}+{{a}^{2}}{{b}^{2}}+{{b}^{4}}}{{{b}^{4}}+{{b}^{2}}{{c}^{2}}+{{c}^{4}}}\]

\[=\frac{{{a}^{4}}+{{a}^{2}}ac+{{a}^{2}}{{c}^{2}}}{{{a}^{2}}{{c}^{2}}+ac{{c}^{2}}+{{c}^{4}}}\]

\[=\frac{{{a}^{2}}\left( {{a}^{2}}+ac+{{c}^{2}} \right)}{{{c}^{2}}\left( {{a}^{2}}+ac+{{c}^{2}} \right)}\]

\[=\frac{{{a}^{2}}}{{{c}^{2}}}=R.H.S.\]

Example 05

If a, b, c are in continued proportion, prove that (a + b +c) (a – b + c) = a² + b² + c².

Answer:

Since a, b, c are in continued proportion

\[\frac{a}{b}=\frac{b}{c}=k(let)\]

\[\Rightarrow b=ck~~and~~a=bk=c{{k}^{2}}\]

\[L.H.S=\left( a+b+c \right)\left( a-b+c \right)\]

\[=\left( c{{k}^{2}}+ck+c \right)\left( c{{k}^{2}}-ck+c \right)\]

\[={{c}^{2}}\left( {{k}^{2}}+k+1 \right)\left( {{k}^{2}}-k+1 \right)\]

\[={{c}^{2}}\left[ {{\left( {{k}^{2}}+1 \right)}^{2}}-{{\left( k \right)}^{2}} \right]\]

\[={{c}^{2}}\left[ {{k}^{4}}+2{{k}^{2}}+1-{{k}^{2}} \right]\]

\[={{c}^{2}}\left[ {{k}^{4}}+{{k}^{2}}+1 \right]\]

\[R.H.S={{a}^{2}}+{{b}^{2}}+{{c}^{2}}\]

\[={{\left( c{{k}^{2}} \right)}^{2}}+{{\left( ck \right)}^{2}}+{{c}^{2}}\]

\[={{c}^{2}}{{k}^{4}}+{{c}^{2}}{{k}^{2}}+{{c}^{2}}\]

\[={{c}^{2}}\left[ {{k}^{4}}+{{k}^{2}}+1 \right]\]

\[\therefore L.H.S=R.H.S\]

Example 06

If (x – 9):(3x + 6) is the duplicate ratio of 4:9, find the value of x.

Answer:

Given: (x – 9):(3x + 6) is the duplicate ratio of 4:9

\[\Rightarrow \frac{x-9}{3x+6}={{\left( \frac{4}{9} \right)}^{2}}\]

\[\Rightarrow \frac{x-9}{3x+6}=\frac{16}{81}\]

\[\Rightarrow 81x-729=48x+96\]

\[\Rightarrow 81x-48x=96+729\]

\[\Rightarrow 33x=825\]

Example 07

\[If~x,~~y,~~z~~are~~in~~continued~~proportion,~~prove~~that~~\frac{{{\left( x+y \right)}^{2}}}{{{\left( y+z \right)}^{2}}}=\frac{x}{z}.~~\]

Answer:

If x, y, z are in continued proportion

\[\frac{x}{y}=\frac{y}{z}=k\]

\[\Rightarrow x=yk~~and~~y=zk\]

\[\Rightarrow x=z{{k}^{2}}~~and~~y=zk\]

\[L.H.S.=\frac{{{\left( x+y \right)}^{2}}}{{{\left( y+z \right)}^{2}}}\]

\[=\frac{{{\left( z{{k}^{2}}+zk \right)}^{2}}}{{{\left( zk+z \right)}^{2}}}\]

\[=\frac{{{z}^{2}}{{k}^{2}}{{\left( k+1 \right)}^{2}}}{{{z}^{2}}{{\left( k+1 \right)}^{2}}}\]

\[={{k}^{2}}\]

\[R.H.S.=\frac{x}{z}=\frac{z{{k}^{2}}}{z}={{k}^{2}}\]

\[Hence,~~L.H.S.=R.H.S.\]

\[\therefore x=25\]

Example 08

\[If~~\frac{{{x}^{2}}+{{y}^{2}}}{{{x}^{2}}-{{y}^{2}}}=\frac{17}{8},~~then~~find~~the~~value~~of:\]

\[(i)~~x:y\]

\[(ii)\frac{{{x}^{3}}+{{y}^{3}}}{{{x}^{3}}-{{y}^{3}}}\]

Answer:

Given,

\[\frac{{{x}^{2}}+{{y}^{2}}}{{{x}^{2}}-{{y}^{2}}}=\frac{17}{8}\]

Applying componendo and dividendo,

\[\Rightarrow \frac{\left( {{x}^{2}}+{{y}^{2}} \right)+\left( {{x}^{2}}-{{y}^{2}} \right)}{\left( {{x}^{2}}+{{y}^{2}} \right)-\left( {{x}^{2}}-{{y}^{2}} \right)}=\frac{17+8}{17-8}\]

\[\Rightarrow \frac{2{{x}^{2}}}{2{{y}^{2}}}=\frac{25}{9}\]

\[\Rightarrow \frac{{{x}^{2}}}{{{y}^{2}}}=\frac{25}{9}\]

\[\Rightarrow \frac{x}{y}=\frac{5}{3}\]

\[\therefore x:y=5:3\]

As, \[x:y=5:3\]

Cubing both sides we get

\[\frac{{{x}^{3}}}{{{y}^{3}}}=\frac{{{5}^{3}}}{{{3}^{3}}}=\frac{125}{27}\]

Applying componendo and dividendo

\[\frac{{{x}^{3}}+{{y}^{3}}}{{{x}^{3}}-{{y}^{3}}}=\frac{125+27}{125-27}\]

\[\Rightarrow \frac{{{x}^{3}}+{{y}^{3}}}{{{x}^{3}}-{{y}^{3}}}=\frac{152}{98}=\frac{76}{49}\]

Example 09

\[If~~x=\frac{\sqrt{a+3b}+\sqrt{a-3b}}{\sqrt{a+3b}-\sqrt{a-3b}},~~prove~~that~~3b{{x}^{2}}-2ax+3b=0\]

Answer:

Given,

\[\frac{x}{1}=\frac{\sqrt{a+3b}+\sqrt{a-3b}}{\sqrt{a+3b}-\sqrt{a-3b}}\]

Applying componendo and dividendo

\[\Rightarrow \frac{x+1}{x-1}=\frac{\sqrt{a+3b}+\sqrt{a-3b}+\sqrt{a+3b}-\sqrt{a-3b}}{\sqrt{a+3b}+\sqrt{a-3b}-\sqrt{a+3b}+\sqrt{a-3b}}\]

\[\Rightarrow \frac{x+1}{x-1}=\frac{2\sqrt{a+3b}}{2\sqrt{a-3b}}=\frac{\sqrt{a+3b}}{\sqrt{a-3b}}\]

Squaring both sides we get,

\[\Rightarrow \frac{{{x}^{2}}+2x+1}{{{x}^{2}}-2x+1}=\frac{a+3b}{a-3b}\]

Applying componendo and dividendo

\[\Rightarrow \frac{\left( {{x}^{2}}+2x+1 \right)+\left( {{x}^{2}}-2x+1 \right)}{\left( {{x}^{2}}+2x+1 \right)-\left( {{x}^{2}}-2x+1 \right)}=\frac{\left( a+3b \right)+\left( a-3b \right)}{\left( a+3b \right)-\left( a-3b \right)}\]

\[\Rightarrow \frac{2{{x}^{2}}+2}{4x}=\frac{2a}{6b}\]

\[\Rightarrow \frac{{{x}^{2}}+1}{2x}=\frac{a}{3b}\]

\[\Rightarrow 3b{{x}^{2}}+3b=2ax\]

\[\Rightarrow 3b{{x}^{2}}-2ax+3b=0\]

Example 10

\[If~~\frac{8a-5b}{8c-5d}=\frac{8a+5b}{8c+5d},~~prove~~that~~\frac{a}{b}=\frac{c}{d}\]

Answer:

Given,

\[\frac{8a+5b}{8c+5d}=\frac{8a-5b}{8c-5d}\]

\[\Rightarrow \frac{8a+5b}{8a-5b}=\frac{8c+5d}{8c-5d}\]

Applying componendo and dividendo

\[\Rightarrow \frac{\left( 8a+5b \right)+\left( 8a-5b \right)}{\left( 8a+5b \right)-\left( 8a-5b \right)}=\frac{\left( 8c+5d \right)+\left( 8c-5d \right)}{\left( 8c+5d \right)-\left( 8c-5d \right)}\]

\[\Rightarrow \frac{16a}{10b}=\frac{16c}{10d}\]

\[\Rightarrow \frac{a}{b}=\frac{c}{d}\]

Example 11

\[Given~~that~~\frac{{{a}^{3}}+3a{{b}^{2}}}{{{b}^{3}}+3{{a}^{2}}b}=\frac{63}{62}.~~\text{Using}~~componendo~~and~~dividendo~~find~~a:b\]

Answer:

Given that,

\[\frac{{{a}^{3}}+3a{{b}^{2}}}{{{b}^{3}}+3{{a}^{2}}b}=\frac{63}{62}\]

By componendo and dividendo

\[\Rightarrow \frac{\left( {{a}^{3}}+3a{{b}^{2}} \right)+\left( {{b}^{3}}+3{{a}^{2}}b \right)}{\left( {{a}^{3}}+3a{{b}^{2}} \right)-\left( {{b}^{3}}+3{{a}^{2}}b \right)}=\frac{63+62}{63-62}\]

\[\Rightarrow \frac{{{a}^{3}}+3a{{b}^{2}}+3{{a}^{2}}b+{{b}^{3}}}{{{a}^{3}}+3a{{b}^{2}}-3{{a}^{2}}b-{{b}^{3}}}=\frac{125}{1}\]

\[\Rightarrow \frac{{{\left( a+b \right)}^{3}}}{{{\left( a-b \right)}^{3}}}={{\left( \frac{5}{1} \right)}^{3}}\]

\[\Rightarrow \frac{a+b}{a-b}=\frac{5}{1}\]

Again applying componendo and dividendo

\[\Rightarrow \frac{a+b+a-b}{a+b-a+b}=\frac{5+1}{5-1}\]

\[\Rightarrow \frac{2a}{2b}=\frac{6}{4}\]

\[\Rightarrow a:b=3:2\]

Example 12

Using componendo and dividendo, find the value of x

\[\frac{\sqrt{3x+4}+\sqrt{3x-5}}{\sqrt{3x+4}-\sqrt{3x-5}}=9\]

Answer:

Given,

\[\frac{\sqrt{3x+4}+\sqrt{3x-5}}{\sqrt{3x+4}-\sqrt{3x-5}}=\frac{9}{1}\]

Applying componendo and dividendo,

\[\Rightarrow \frac{\sqrt{3x+4}+\sqrt{3x-5}+\sqrt{3x+4}-\sqrt{3x-5}}{\sqrt{3x+4}+\sqrt{3x-5}-\sqrt{3x+4}+\sqrt{3x-5}}=\frac{9+1}{9-1}\]

\[\Rightarrow \frac{2\sqrt{3x+4}}{2\sqrt{3x-5}}=\frac{10}{8}\]

\[\Rightarrow \frac{\sqrt{3x+4}}{\sqrt{3x-5}}=\frac{5}{4}\]

Squaring both sides,

\[\Rightarrow \frac{3x+4}{3x-5}=\frac{25}{16}\]

Again applying componendo and dividendo,

\[\Rightarrow \frac{3x+4+3x-5}{3x+4-3x+5}=\frac{25+16}{25-16}\]

\[\Rightarrow \frac{6x-1}{9}=\frac{41}{9}\]

\[\Rightarrow 6x-1=41\]

\[\Rightarrow 6x=42\Rightarrow x=7\]

Example 13

\[Given~~\frac{{{x}^{3}}+12x}{6{{x}^{2}}+8}=\frac{{{y}^{3}}+27y}{9{{y}^{2}}+27}\]

Using componendo and dividendo find x:y

Answer:

Solve yourself.

Example 14

\[If~~\frac{x}{a}=\frac{y}{b}=\frac{z}{c},~~show~~that~~\frac{{{x}^{3}}}{{{a}^{3}}}+\frac{{{y}^{3}}}{{{b}^{3}}}+\frac{{{z}^{3}}}{{{c}^{3}}}=\frac{3xyz}{abc}.\]

Answer:

\[Given:~~\frac{x}{a}=\frac{y}{b}=\frac{z}{c}=k(say)\]

\[\Rightarrow x=ak,~~y=bk,~~z=ck\]

\[L.H.S=\frac{{{x}^{3}}}{{{a}^{3}}}+\frac{{{y}^{3}}}{{{b}^{3}}}+\frac{{{z}^{3}}}{{{c}^{3}}}\]

\[=\frac{{{a}^{3}}{{k}^{3}}}{{{a}^{3}}}+\frac{{{b}^{3}}{{k}^{3}}}{{{b}^{3}}}+\frac{{{c}^{3}}{{k}^{3}}}{{{c}^{3}}}\]

\[={{k}^{3}}+{{k}^{3}}+{{k}^{3}}\]

\[=3{{k}^{3}}\]

\[=3k.k.k\]

\[=3\frac{x}{a}.\frac{y}{b}.\frac{z}{c}\]

\[=\frac{3xyz}{abc}=R.H.S.\]

Example 15

Using properties of proportion, solve for x. Given that x is positive:

\[\frac{2x+\sqrt{4{{x}^{2}}-1}}{2x-\sqrt{4{{x}^{2}}-1}}=4\]

Answer:

\[Given,\frac{2x+\sqrt{4{{x}^{2}}-1}}{2x-\sqrt{4{{x}^{2}}-1}}=4\]

By componendo and dividendo, we have

\[\frac{2x+\sqrt{4{{x}^{2}}-1}+2x-\sqrt{4{{x}^{2}}-1}}{2x+\sqrt{4{{x}^{2}}-1}-2x+\sqrt{4{{x}^{2}}-1}}=\frac{4+1}{4-1}\]

\[\Rightarrow \frac{4x}{2\sqrt{4{{x}^{2}}-1}}=\frac{5}{3}\]

\[\Rightarrow \frac{2x}{\sqrt{4{{x}^{2}}-1}}=\frac{5}{3}\]

Squaring both sides, we have

\[\frac{4{{x}^{2}}}{4{{x}^{2}}-1}=\frac{25}{9}\]

\[\Rightarrow 100{{x}^{2}}-25=36{{x}^{2}}\]

\[\Rightarrow 100{{x}^{2}}-36{{x}^{2}}=25\]

\[\Rightarrow 64{{x}^{2}}=25\]

\[\Rightarrow {{x}^{2}}=\frac{25}{64}\]

\[\therefore x=\frac{5}{8}~~\left[ \because ~~x~~is~~positive \right]\]

Next Topic >> Laws of Indices

;