Equation of a Straight Line
We know that the common section of two planes is a straight line. So now, let’s talk about the Equation of a Straight Line. Let the equations of the two non-parallel planes be ax + by + cz + d = 0 and a’x + b’y + c’z + d’ = 0.
Let AB be the line of intersection of the two planes. Every point on the straight line AB lies on both the planes and the co-ordinates of any point on the line satisfy the two equations of the planes. Hence the straight line is represented by two first degree equations in x, y, z. Though each equation represents a plane, both together represent the straight line which is the common section of the two planes.
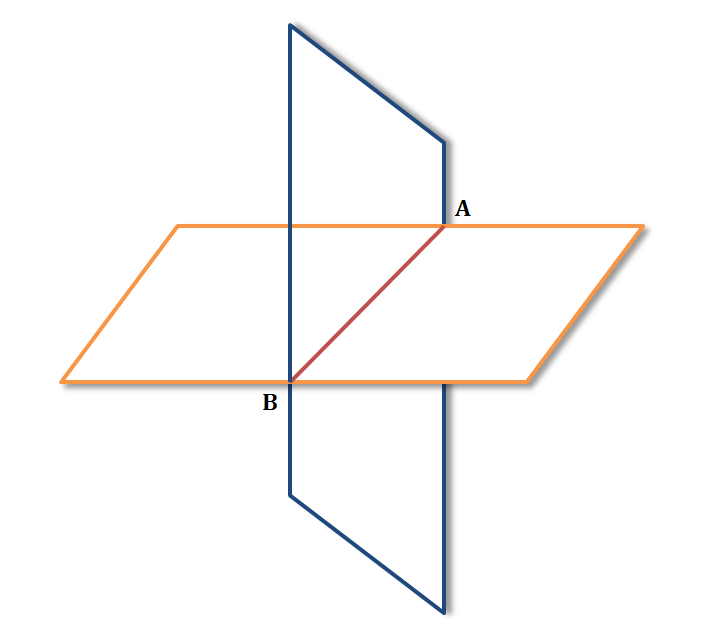
The equations ax + by + cz = 0 and a’x + b’y + c’z = 0 together represent the straight line through the origin parallel to the above straight line.
The above form of the equation of a straight line is referred to as non-symmetrical form.
Cor. The x-axis is the intersection of xy-plane and xz-plane, that is, of z = 0 and y = 0 planes. Hence the equations of the x-axis are y = 0 = z. Similarly the equations of y-axis and z-axis are respectively x = 0 = z and x = 0 = y.
Some Formulae on Straight Line
1. If the line passes through the point (x1, y1, z1) and its direction ratios are l, m, n then the equations of the line are
\[\frac{x-{{x}_{1}}}{l}=\frac{y-{{y}_{1}}}{m}=\frac{z-{{z}_{1}}}{n}\]
2. If the line passes through two given points (x1, y1, z1) and (x2, y2, z2) then its equations are
\[\frac{x-{{x}_{1}}}{{{x}_{2}}-{{x}_{1}}}=\frac{y-{{y}_{1}}}{{{y}_{2}}-{{y}_{1}}}=\frac{z-{{z}_{1}}}{{{z}_{2}}-{{z}_{1}}}\]
3. Parametric Form: If the line passes through the point (x1, y1, z1) and direction ratios be l, m, n then its equations are x=lr+{{x}_{1}},\,\,y=mr+{{y}_{1}},\,\,z=nr+{{z}_{1}} where r is the parameter.
4. Plane Intercept Form: The planes ax + by + cz + d = 0 and a’x + b’y + c’z + d’ = 0 together represent the straight line of intersection of the planes.
5. Coplanarity of two Straight lines: The lines \frac{x-{{x}_{1}}}{{{l}_{1}}}=\frac{y-{{y}_{1}}}{{{m}_{1}}}=\frac{z-{{z}_{1}}}{{{n}_{1}}} and \frac{x-{{x}_{2}}}{{{l}_{2}}}=\frac{y-{{y}_{2}}}{{{m}_{2}}}=\frac{z-{{z}_{2}}}{{{n}_{2}}} are coplanar iff
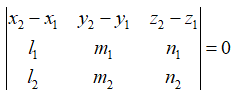
6. Perpendicular distance of a point from a line: The perpendicular distance from the point (α, β, γ) to the line \frac{x-{{x}_{1}}}{l}=\frac{y-{{y}_{1}}}{m}=\frac{z-{{z}_{1}}}{n} is
\[{{\left[ {{\left( \alpha -{{x}_{1}} \right)}^{2}}+{{\left( \beta -{{y}_{1}} \right)}^{2}}+{{\left( \gamma -{{z}_{1}} \right)}^{2}}-\frac{{{\left\{ l\left( \alpha -{{x}_{1}} \right)+m\left( \beta -{{y}_{1}} \right)+n\left( \gamma -{{z}_{1}} \right) \right\}}^{2}}}{{{l}^{2}}+{{m}^{2}}+{{n}^{2}}} \right]}^{\frac{1}{2}}}\]
7. Shortest distance between two Skew Lines: The shortest distance between lines
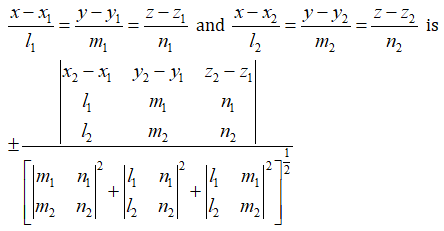
| Example 01 |
Show that the direction cosines of the straight line x + y – z + 1 = 0, 4x + y – 2z + 2 =0 are \left( \pm \frac{1}{\sqrt{14}},\pm \frac{2}{\sqrt{14}},\pm \frac{3}{\sqrt{14}} \right).
Solution:
Let l, m, n be the direction ratios of the straight line
\[\therefore \,\,l+m-n=0\,\,and\,\,4l+m-2n=0\]
By cross-multiplication, we have
\[\frac{l}{-2+1}=\frac{m}{-4+2}=\frac{n}{1-4}\]
\[\Rightarrow \frac{l}{1}=\frac{m}{2}=\frac{n}{3}\]
Therefore the direction cosines are
\[\pm \frac{1}{\sqrt{1+4+9}},\pm \frac{2}{\sqrt{1+4+9}},\pm \frac{3}{\sqrt{1+4+9}}\]
\[i.e.,\,\,\pm \frac{1}{\sqrt{14}},\pm \frac{2}{\sqrt{14}},\pm \frac{3}{\sqrt{14}}\]
| Example 02 |
Show that the equation of a straight line through the point (3, 4, 5) which is equally inclined to the axes are x-3=y-4=z-5.
Solution:
If \cos \alpha ,\,\cos \beta ,\,\cos \gamma be the direction cosines of the straight line, then \alpha =\beta =\gamma
\[i.e.,\,\cos \alpha =\,\cos \beta =\,\cos \gamma \]
Therefore the equations of the line are
\[\frac{x-3}{\cos \alpha }=\frac{y-4}{\cos \beta }=\frac{z-5}{\cos \gamma }\]
\[i.e.,\,\,x-3=y-4=z-5\]
| Example 03 |
Put the equation of a straight lines in symmetrical from as given by
\[x+5y-z-7=0,\,\,2x-5y+3z+1=0\]
Solution:
Let the direction ratios of the line be l, m, n
\[\therefore \,\,l+5m-n=0,\,\,2l-5m+3n=0\]
By cross-multiplication, we have
\[\frac{l}{15-5}=\frac{m}{-2-3}=\frac{n}{-5-10}\]
\[\Rightarrow \frac{l}{2}=\frac{m}{-1}=\frac{n}{-3}\]
The point where the line meets the plane z = 0 is given by x+5y=7\,\,and\,\,2x-5y+1=0.
Solving, x=2,\,\,y=1
Thus one point on the line is (2, 1, 0).
Therefore the equations of the line in symmetrical form are
\[\frac{x-2}{2}=\frac{y-1}{-1}=\frac{z-0}{-3}\]
| Example 04 |
Find the image of the straight line \frac{x-1}{3}=\frac{y-3}{5}=\frac{z-4}{2} in the plane 2x-y+z+3=0.
Solution:
The given equation of a straight line is
\[\frac{x-1}{3}=\frac{y-3}{5}=\frac{z-4}{2}=r\,\left( say \right)\]
Then any point on it is given by x=3r+1,\,y=5r+3,\,z=2r+4
If this point lies on the given plane 2x-y+z+3=0. Then
\[2\left( 3r+1 \right)-\left( 5r+3 \right)+\left( 2r+4 \right)+3=0\]
\[\Rightarrow 6r+2-5r-3+2r+4+3=0\]
\[\Rightarrow \,\,r=-2\]
So, the point is (–5, –7, 0).
Let B be the image of point A(1, 3, 4) in the plane. The equation of a straight line AB is
\[\frac{x-1}{2}=\frac{y-3}{-1}=\frac{z-4}{1}=R\,\left( say \right)\]
Let the co-ordinates of B be \left( 2R+1,\,\,-R+3,\,R+4 \right).
The co-ordinates of P, the middle point of AB, are then \left( R+1,-\frac{R}{2}+3,\frac{R}{2}+4 \right)
Now, since P lies on the plane 2x-y+z+3=0, we have
\[2\left( R+1 \right)-\left( 3-\frac{R}{2} \right)+\left( \frac{R}{2}+4 \right)+3=0\]
\[\Rightarrow 2R+2-3+\frac{R}{2}+\frac{R}{2}+4+3=0\]
\[\Rightarrow R=-2\]
Hence B, the image of A, is the point (–3, 5, 2).
Thus the image of the straight line joining (–5, –7, 0) and (–3, 5, 2) is
\[\frac{x+3}{-3+5}=\frac{y-5}{5+7}=\frac{z-2}{2-0}\]
\[\Rightarrow \,\frac{x+3}{2}=\frac{y-5}{12}=\frac{z-2}{2}\]
\[i.e.,\,\frac{x+3}{1}=\frac{y-5}{6}=\frac{z-2}{1}\]
| Example 05 |
Find the equations of a straight line through the point (8, 9, –10) and perpendicular to each of the straight lines \frac{x-2}{3}=\frac{y-3}{2}=\frac{z-4}{4}\,\,and\,\,\frac{x+1}{5}=\frac{y-2}{-6}=\frac{z+3}{2}
Solution:
Any line through the point (8, 9, –10) is
\[\frac{x-8}{l}=\frac{y-9}{m}=\frac{z+10}{n}\]
Where l, m, n are the direction cosines of the line, this line is perpendicular to the given lines
\[\therefore 3l+2m+4n=0\,\,and\,\,5l-6m+2n=0\]
By cross-multiplication, we have
\[\frac{l}{4+24}=\frac{m}{20-6}=\frac{n}{-18-10}\]
\[\Rightarrow \frac{l}{28}=\frac{m}{14}=\frac{n}{-28}\]
\[\Rightarrow \frac{l}{2}=\frac{m}{1}=\frac{n}{-2}\]
Hence the required equation of the line is
\[\frac{x-8}{2}=\frac{y-9}{1}=\frac{z+10}{-2}\]
| Example 06 |
Find the equation of a straight line through the point (1, 2, 3) and parallel to the straight line joining the points (–4, 7, 2) and (5, –3, –2).
Solution:
Let the equation of a straight line through (1, 2, 3) be
\[\frac{x-1}{l}=\frac{y-2}{m}=\frac{z-3}{n}\,\,………\left( 1 \right)\]
The equation of the line joining the points (–4, 7, 2) and (5, –3, –2)
\[\frac{x+4}{5+4}=\frac{y-7}{-3-7}=\frac{z-2}{-2-2}\,\]
\[\Rightarrow \frac{x+4}{9}=\frac{y-7}{-10}=\frac{z-2}{-4}\,\,………\left( 2 \right)\,\]
Since the line (2) is parallel to (1) then
\[\frac{l}{9}=\frac{m}{-10}=\frac{n}{-4}\]
\[\Rightarrow \frac{l}{-9}=\frac{m}{10}=\frac{n}{4}=k\,\left( say \right)\]
\[\therefore \,\,l=-9k,\,\,m=10k,\,\,n=4k\]
Putting the values of l, m, n in (1) we get the required equation
\[\frac{x-1}{-9}=\frac{y-2}{10}=\frac{z-3}{4}\]
Plane |
Sphere |


